Linear and angular displacement, velocity and acceleration

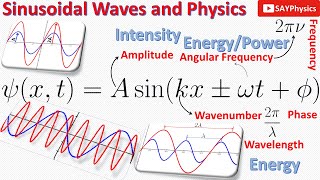
Now, we consider a circular motion in which $$ d=2r $$As we calculated that $$ S=2\pi r=\pi d $$ which I call the circumference, so I can write that $$ \pi =\frac { D }{ d } =3.1416...\sim \frac { 22 }{ 7 } $$ It is basically the ratio of the circumference with the diameter. Now the angular displacement we will have to measure which can be measured in radians and degrees. Now, we know that radians and degrees are two measurements, the circle completes by going 2? radians and in degrees 360 degrees. So, from here I can write that $$ 2\pi \text{ radians }={ 360 }^{ o } $$ and I can write further that $$ 1 \text{ radian }=\frac { { 360 }^{ o } }{ 2\pi } $$ Now as I have written earlier that $$ S=r\theta \Rightarrow \theta =\frac { S }{ r } =\frac { \text { arc length } }{ \text { radius } } =1 \text { rad } $$ When the arc length is exactly equal to the radius then the angle will be actually equal to one radian. Now one radian is equal to how many degrees? $$ 2\pi \text { radians } ={ 360 }^{ o } $$ $$ 1 \text { rad } = { 57.29 }^{ o } $$ The linear displacement is \(x\) while the angular displacement is \(\theta \), these two are related with each other $$ S=r\theta $$ Now, when this displacement will change in time, then we define another thing, called velocity. What is velocity? Velocity is the rate of change of displacement. $$ \frac { \Delta x }{ \Delta t } \equiv v $$ And this will be measured in meter. This I call the linear velocity, now what about the angular velocity? The angular velocity is a body moving in a circle then it is covering angular displacement which is \(theta \), the angular velocity is the rate of change of angular displacement and we will denote this angular velocity by \(\omega \). $$ \frac { \Delta \theta }{ \Delta t } \equiv \omega $$ To establish the relation between the linear velocity, \(v\) and angular velocity \( \omega \) $$ \frac { \Delta \theta }{ \Delta t } =\omega =\frac { \theta }{ t } =\frac { S }{ r } \frac { 1 }{ t } $$ From this expression I can write $$ \omega =v\frac { 1 }{ r } \Rightarrow v=r\omega $$ and $$\omega =\frac { rad }{ s } $$ Now we discuss acceleration. Acceleration is the rate of change of velocity, which means how much the velocity is changing with respect to time $$ a\equiv \frac { \Delta v }{ \Delta t } $$ This is the linear acceleration and the angular acceleration is, when a body accelerates in circular motion. $$ \alpha \equiv \frac { \Delta \omega }{ \Delta t } $$ Let's derive the relation between linear and angular acceleration. $$ \alpha =\frac { \omega }{ t } =\frac { v }{ r } \frac { 1 }{ t } =\frac { a }{ r } $$ So from here I can write that $$ a=r\alpha $$ $$ v=r\omega $$ $$ s=r\theta $$ So this way we are having our symmetries between the angular and the linear physical quantities. Now let me write \( \alpha \) in terms of the displacement $$ a=0 $$ $$ \frac { dv }{ dt } =0 $$ If I integrate both sides, I will get $$ v= \text { constant } $$ This means that when linear acceleration is zero, we are having a constant linear velocity. Similarly, now come back to the \( \alpha \), then $$ \alpha =\frac { d\omega }{ dt } =\frac { d }{ dt } \frac { d\theta }{ dt } \frac { { d }^{ 2 }\theta }{ d{ t }^{ 2 } } $$ When \( \alpha = 0 \) $$ \frac { d\omega }{ dt } =0 $$ $$ \omega = \text { constant } $$ This means that when angular acceleration is zero, we are having a constant angular velocity. The unit of acceleration is \(\frac { m }{ { s }^{ 2 } } \) and that of angular acceleration is \(\frac { rad }{ { s }^{ 2 } } \)