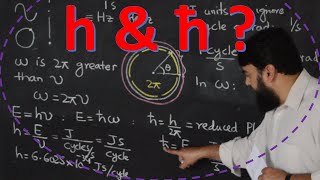Difference between linear frequency and angular frequency

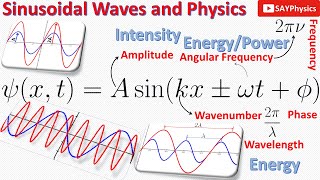
We will discuss the difference between linear frequency and angular frequency. We define linear frequency as the number of cycles which are passing through a certain point in a unit time which is one second. $$\text { Linear frequency } = \frac { \text { no. of cycles } }{ \text { time } }$$ and we define this in units of Hertz, so how many cycles per second are there is called Hertz. $$v = \frac { cycles }{ 1s } =Hz=Hertz$$ Angular frequency means how many rotations are completed or how many cycles are completed in one second. So, I can write that $$\omega =\frac { \text { no. of revolutions } }{ s } =\frac { rad }{ s }$$ The angular frequency or the angular velocity is actually the same thing. We define this in radians per second and sometimes in SI units we ignore the word cycle or radians and we only call it per second, this create a confusion between the linear dynamics and the angular dynamics. Let's consider a circle and let's say we are having $$\frac { 1 cycle }{ s } =2\pi rad=1Hz$$ In angular frequency we will call this $$\frac { 1\quad rad }{ s }$$ Now one radian here is actually not $2 \pi$ but one radian, as we discussed in earlier lectures, that one radian is the angle in which the arc length becomes equal to the radius of the circle, so I can write that, $\omega$ is $2 \pi$ greater than $v$ $$\omega =2\pi v$$