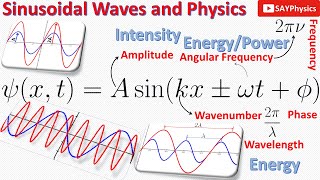
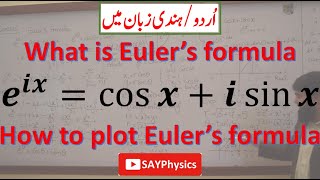
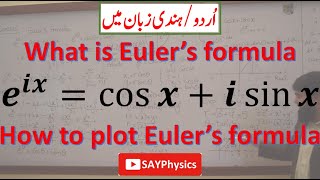
Conceptual Physics: Hilbert space vs vector space in simple words

First let's talk about the vector space, it is a three-dimensional space x, y and z. We call the unit vectors. What are their properties? The properties are if you take the magnitude of these, which will be equal to 1. If you need the dot product of each of them with each other then they will be equal to 0. Why? Because they are perpendicular to each other. This condition shows orthogonally and can mean that these unit vectors are normalized. The unit vectors are orthonormal in nature. $$ \left| \hat { x } \right| =\left| \hat { y } \right| =\left| \hat { z } \right| =1 $$ $$ \hat { x } .\hat { y } =\hat { y } .\hat { z } =\hat { z } .\hat { x } =0 $$Instead of unit vectors I want to give it a new name which is bases of vector space. Any vector which I define in this space can be resolved in components of these bases. $$ \vec { r } =x.\hat { x } +y.\hat { y } +z.\hat { z } $$Here now this x is the magnitude or the coefficient or the portion of this r which is along x. Sometimes instead of \(xyz\), you write \(ijk\) which are orthonormal bases, or in a different notation like: $$ \vec { r } =x.\hat { i } +y.\hat { j } +z.\hat { k } $$ $$ \vec { r } =x.\hat { { e }_{ x } } +y.\hat { { e }_{ y } } +z.\hat { { e }_{ z } } $$ So this is a vector three-dimensional space, whenever the bases qualify to be orthonormal, then you can expand any vector in terms of them.

Now let's discuss the Hilbert's space, then we will compare different parameters. In a vector space you can resolve any of its components, but a Hilbert's space is not dealing with a vector. It deals with functions. There are only 3 components in vector space but a function have infinite number of components or n-components. How many orthonormal bases we should have? Again n-number of bases we should have. If $$ { \psi }_{ 1 }\left( x \right) ,{ \psi }_{ 2 }\left( x \right) ,{ \psi }_{ 3 }\left( x \right) ,...,{ \psi }_{ n }\left( x \right) $$ are orthonormal, we can say that these are the components of Hilbert's space, but these will follow some conditions which are: They are supposed to be orthonormal $$\int { { \psi }_{ m }*\left( x \right) { \psi }_{ n }\left( x \right) dx } ={ \delta }_{ mn } $$ As the Hilbert's space is n-dimensional, its components can be written as $$ \psi \left( x \right) =\sum _{ n=1 }^{ \infty }{ { C }_{ n }{ \psi }_{ n }\left( x \right) } $$ This can be expanded as: $$\psi \left( x \right) ={ c }_{ 1 }{ \psi }_{ 1 }\left( x \right) +{ c }_{ 2 }{ \psi }_{ 2 }\left( x \right) +{ c }_{ 3 }{ \psi }_{ 3 }\left( x \right) +...$$ Now what if we want to find a specific component say \(c_1\)? We'll use Fourier trick $$ { c }_{ 1 }=\int { { \psi }_{ 1 }*\left( x \right) \psi \left( x \right) dx } ={ c }_{ 1 }$$ $$ { c }_{ n }=\int _{ -\infty }^{ +\infty }{ { \psi }_{ n }*\left( x \right) f\left( x \right) dx } $$In vector space, we can find the magnitude of r as: $$ \vec { r } =\sqrt { { x }^{ 2 }+{ y }^{ 2 }+{ z }^{ 2 } } =1 $$ In Hilbert's space, $$ \sum _{ n=1 }^{ \infty }{ { \left| { c }_{ n } \right| }^{ 2 } } =1 $$ This \(c_n\) is actually the x, y, z magnitudes in vector space. You can treat your vector in Hilbert's space as it is having many components but you cannot treat a function in vector space as it has only three components.