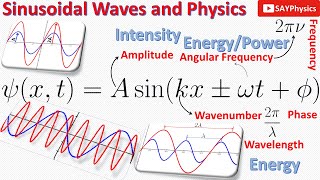
Conceptual Physics: Basics of sinusoidal waves and its properties II

So energy of the individual photon is changed only when the source is changed to a high energy source and now we will explain the next term in the equation $$ \Psi \left( x,t \right) =A\sin { \left( kx\pm \omega t+\varphi \right) } $$ which is actually the wave number. The wave number is defined as $$ \frac { 2\pi }{ \lambda } $$ where \(2 \pi \) is actually considered as a unit angular distance which is \(2 \pi \) radians. Wavelength is the distance over which a wave repeats a shape. We know that energy is inversely proportional to the wavelength because energy is equal to $$ E=hv=\frac { hc }{ \lambda } $$ The shorter the wavelength the high will be the energy and the longer the wavelength the less will be the energy. Wavelength is inversely proportional to the energy and now the important one is the wave number. Wave number is defined as the number of cycles in a unit distance, ? is the angular frequency which is equal to $$ \omega =2\pi v $$ where \(v \) is the frequency. As we know that in science we define physical quantities in terms of space and time. So if I want to see the number of waves in a given distance then I will call it wave number and it will be $$ \frac { 1 }{ m } ={ m }^{ -1 } $$ And if I want to see the number of waves in a unit time then it will be $$\frac { 1 }{ t } ={ s }^{ -1 } $$ Or per second which we call Hertz as well. So simply we see that wave number is the waves per distance while frequency which we call the time frequency are the waves per time.

Now the big question is why we need a wave number if wavelength is there which is inversely proportional and frequency is there which is directly proportional to the energy? As we know that $$ E=hv=\frac { hc }{ \lambda } $$ c being the speed of light $$ \omega =2\pi v=ck $$ and from the dispersion relation I can write that $$ c=v\lambda $$ and from here I can write that $$ k=\frac { 2\pi }{ \lambda } $$In spectroscopy we plot absorption or transmission with respect to different variables for example we plot in UV-vis the absorption with respect to wavelength and it is from lower wavelength to the higher wavelength. Similarly in PL spectroscopy we plot the emission intensity with respect to wavelength and in some cases when we want to calculate the band gap from the UV-vis spectroscopy then we calculate energy and we draw a slope on it.

Why in IR spectra we need the wave number? This is a very important question, in order to understand this thing we take the equation of a harmonic oscillator because we are understanding the oscillations of atom we want to understand in IR spectra the atomic excitations inside a molecule where atoms are attached to each other like two masses attached to a spring. So we use the harmonic oscillator equation $$ F=-Kx $$where K is the spring constant. Now we can write $$ F=ma=\frac { { d }^{ 2 }y }{ d{ t }^{ 2 } } =-Kx $$ From this we can derive that the $$ v=\frac { 1 }{ 2\pi } \sqrt { \frac { K }{ m } } $$ Now I can change this equation by $$ \frac { v }{ c } =k=\frac { 1 }{ 2\pi c } \sqrt { \frac { K }{ m } } $$ then in order to convert the masses into atomic mass unit and the mass is two atoms means I will be discussing the bonds between two atoms so the mass will be replaced by the reduced mass $$ k=\frac { 1 }{ 2\pi c } \sqrt { \frac { K{ N }_{ A } }{ \mu } } $$ $$ \mu =\frac { { m }_{ 1 }+{ m }_{ 2 } }{ { m }_{ 1 }{ m }_{ 2 } } $$ $$ \mu =\left( 2911\sqrt { g } /cm \right) \sqrt { \frac { 1 }{ \mu } } $$ Wave number actually resolves the closely spaced peaks and this is the benefit of doing this.

The last thing is the phase shift. When the starting point of two waves is different, that lag can be defined as phase shift.

What will happen when two or more waves are having different phases? This will cause the interference of waves.

One question is that is the k-space a momentum space? Our k-space is a reciprocal space so the answer to this is, Yes. We talk in solid-state physics in terms of a momentum space or we talk in terms of reciprocal space or reciprocal lattice so this k is actually the same thing but in order to understand this thing the k space or the reciprocal space are the diffraction pattern we get from a material with the help of x-ray.